Wallis

Wallis

‘About the beginning of my mathematical studies, as soon as the works of our celebrated countryman, Dr Wallis, fell into my hands, by considering the Series, by the Intercalation of which, he exhibits the Area of the Circle and the Hyperbola….’

Isaac Newton, Two Treatises of the Quadrature of Curves, p. 355.

John Wallis, Opera Mathematica (Oxford, 1699), portrait.

Although John Wallis (1616-1703) published the first volume of his Opera mathematica in 1695, many of the seven works contained in the volume were written and published separately decades earlier. The first volume of Opera mathematica contained many of the most important contributions Wallis made to seventeenth century mathematics, all of which were written during Wallis’ time as the Savilian Chair of Geometry at Oxford (which he held from 1649 until his death).

The most important two works in the first volume of Opera mathematica were De sectionibus conicis and Arithmetica infinitorum. De sectionibus conicis was originally published in 1655, and in it Wallis proved that conic sections could be defined adequately by just algebraic equations. It was in this work that Wallis first used the ∞ symbol and gave it a meaning in mathematical context.

Arithmetica infinitorum, originally published in 1656, is widely regarded as Wallis’ most influential work. Wallis himself translated the title as ‘Arithmetick of Infinites’, but ‘Arithmetic of infinitesimals’ is likely a more accurate and descriptive translation according to Stedall (2005). Elsewhere, she underscores Wallis’ contribution as follows:

The first writer to work with the concept of a limit in something like the modern sense was Wallis, who in his Arithmetica infinitorum in 1656 repeatedly claimed that two quantities whose difference could be made less than any assignable quantity could ultimately be considered equal.

Jacqueline Stedall, Mathematics Emerging. A Sourcebook 1540-1900, p. 291.

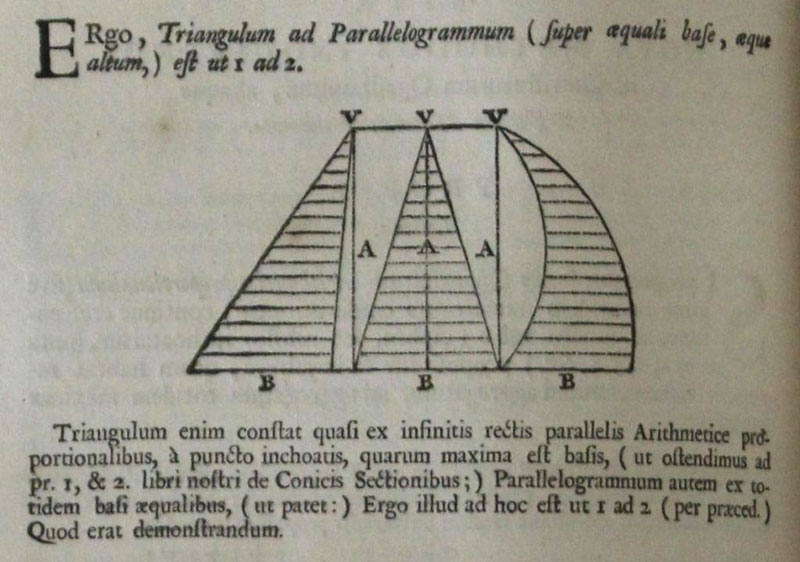
John Wallis, Opera Mathematica (Oxford, 1699), p. 366.

Arithmetica infinitorum was groundbreaking for its time for many reasons, one of which was its inclusion an expression for as an infinite product:
= (3 x 3 x 5 x 5 x 7 x 7 x …)/(2 x 4 x 4 x 6 x 6 x 8 x …)

John Wallis, Opera Mathematica (Oxford, 1699), p. 468.

Throughout much of Opera mathematica, it is apparent that Wallis was inspired by the works of Johannes Kepler (1571-1630), Gilles Personne de Roberval (1602-1675), René Descartes (1596-1650), Bonaventura Cavalieri (1598-1647) and Evangelista Torricelli (1608-1647). This is especially clear in Arithmetica infinitorum, where Wallis drew from Kepler’s idea of continuity and Cavalieri’s method of indivisibles to extend ideas relating to calculus, converting the geometric problem to an algebraic one. It was this approach that gave Arithmetica infinitorum such an important standing at the time of its original publication, evidenced by the fact that Newton cited studying and being inspired by the text while he was a student (see above). Indeed, Wallis is often considered the most influential English mathematician before Newton.

Also included in the first volume of Opera mathematica were Mathesis universalis (Universal Mathematics), De proportionibus dialogum, Eclipsis solaris Observatio, De cycloide Tractatus (Tract on the Cycloid) and Mechanica; sive Tractacus de Motu (Mechanics, or Tract on Motion).

It is not completely clear why Edward Worth had this volume of Opera mathematica in his collection and not the other two volumes, all of which were published in his lifetime. It is possible that Worth obtained volume one precisely because it contained Arithmetica infinitorum, a work that was so influential to Newton, many of whose works Worth collected.

Sources

Anon, ‘John Wallis’ entry in Encyclopædia Britannica (2016).

Clerke, Agnes, ‘John Wallis (1616-1703)’, entry in Dictionary of National Biography, (London, 1899).

Newton, Sir Isaac, Sir Isaac Newton’s Two Treatises of the quadrature of curves, edited by John Stewart (London, 1745).

O’Connor, J.J. and Robertson E.F., John Wallis, MacTutor History of Mathematics, (University of St Andrews, Scotland, February 2002).

Stedall, Jacqueline, ‘John Wallis: Arithmetica Infinitorum’ in Grattan-Guinness, Ivor (ed.), Landmark Writings in Western Mathematics: 1640-1940 (Amsterdam: Elzevier, 2005), 23-32.

Stedhall, Jacqueline, Mathematics Emerging: A Sourcebook 1540-1900 (Oxford University Press, 2008).

Text: Pamela McKee and Maurice OReilly.